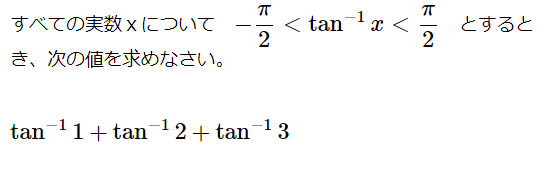ここで勉強すれば数学検定1級の壁は超えられるか。
MENU
数学検定1級の壁 TOP > 数検1級の三角関数 > 逆余弦と逆正接(三角関数2)
逆余弦と逆正接(三角関数2)

tan(cos−1x)=sin(tan−147)
のxを求める。
ただし0≦cos−1x≦π,−π2<tan−1<π2である。
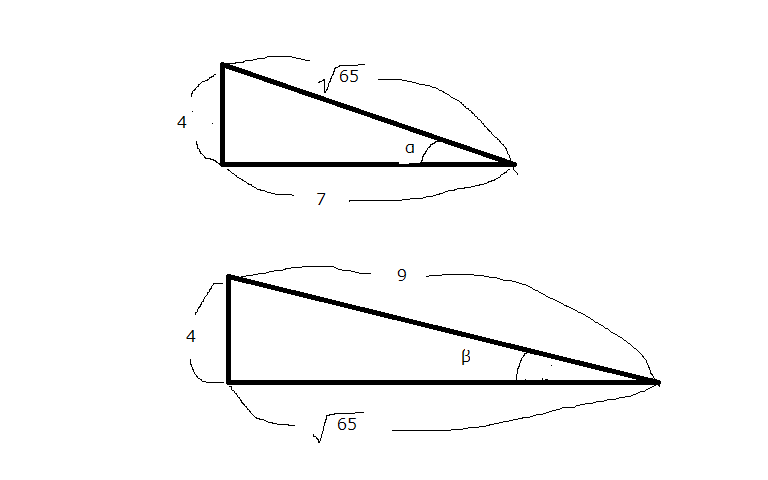
図の上の三角形の比より
tan−147=α→sinα=4√65
図の下の三角形の比より
cos1x=β→tanβ=4√65(0≦β≦π)
したがって
x=cosβ=√659 ・・・答え
同じカテゴリー「数検1級の三角関数」の一覧
サイン4つの積 (三角関数6)
積 [math]\sin 20^{\circ }\cdot \sin 40^{\circ }\cdot \sin 60^{\circ }\cdot \sin 80^{\c […]
逆正接の加法定理 (三角関数5)
a,bを相異なる正の実数とします。このとき [math]\tan ^{-1}\dfrac {a}{b}+\tan ^{-1}\dfrac {a+b}{a-b} […]
加法定理 (三角関数4)
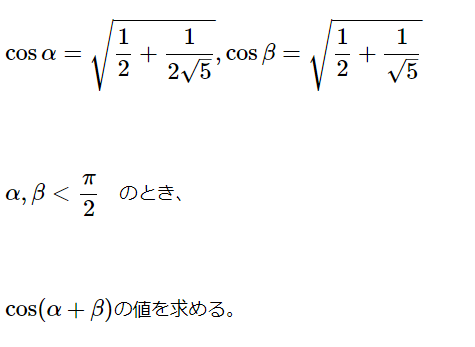
[math]\cos \alpha =\sqrt {\dfrac {1}{2}+\dfrac {1}{2\sqrt {5}}},\cos \beta =\sqrt {\dfrac {1}{2}+\df […]