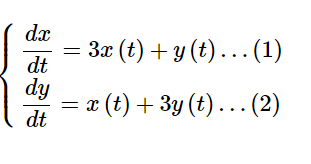微分方程式19の解説
MENU
数学検定1級の壁 TOP > 数検1級の微分方程式 > 微分方程式19の解説
微分方程式19の解説

y(0)=−3のとき次の微分方程式を解きなさい。
dydx=(y−x)2
y−x=uとおいて両辺をⅹで微分すると
dydx−1=dudxとなり、上の微分方程式は
dudx=u2−1となる。
1u2−1du=dxを解いて
∫12{1u−1−1u+1}du=∫dx
logu−1u+1=2x+c cは積分定数
u−1u+1=y−x−1y−x+1=Ae2x・・・(1)
Aは積分定数
y(0)=−3より積分定数A=−3−0−1−3−0+1=2
(1)より
y−x−1=2e2x(y−x+1)
(1−2e2x)y=(1−2e2x)x+1+2e2x
y=x+1+2e2x1−2e2x・・・答
同じカテゴリー「数検1級の微分方程式」の一覧
微分方程式15の解説(連立微分方程式)
次の連立微分方程式を解く。 [math]\begin{cases}\dfrac {dx}{dt}=3x\left( t\right) +y\left( t\right) \ […]