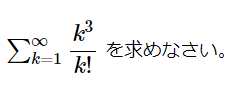ここで勉強すれば数学検定1級の壁は超えられるか。
MENU
数学検定1級の壁 TOP > 数検1級の極限 > 分母の有理化だけで求める(極限4)
分母の有理化だけで求める(極限4)

limx→36−x2+x√x2−89−x2
=limx→3(1+x√x2−8−39−x2)
=limx→3(1+x√x2−8−39−x2⋅x√x2−8+3x√x2−8+3)
=1+limx→3x2(x2−8)−9(9−x2)(x√x2−8+3)
=1+limx→3(x2−9)(x2+1)(9−x2)(x√x2−8+3)
したがって
=1+−( 32+1)3+3=1−106=−23・・・答え
ロピタルの定理でも同様にできる。
同じカテゴリー「数検1級の極限」の一覧
Tanを含む式の極限値(極限15)
[math]\lim _{x\rightarrow +0}\left( \dfrac {\tan x}{x}\right) ^{\dfrac {1}{x^{2}}}[/ma […]
3乗根を含んだ無限級数(極限12)
次の級数が収束するような実数xの値の範囲を求める。 [math]\sum ^{\infty }_{n=1}\dfrac {3^{n}}{\sqrt [3] {n}}x^{n […]