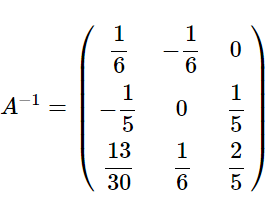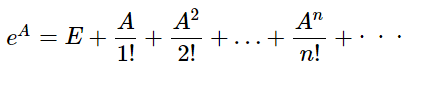ここで勉強すれば数学検定1級の壁は超えられるか。
行列22の解説 (文字式を含んだ3行3列の逆行列)

次の逆行列を求める。ただし、xは0でなく、かつ逆行列をもつような実数とする。
(−11+x1+x−11+x−1−11+x1+x1+x−1−1)
余因子行列を行列式で割って逆行列を求める。
A=(−11+x1+x−11+x−1−11+x1+x1+x−1−1)の逆行列を求める。
|A|=|−11+x1+x−11+x−1−11+x1+x1+x−1−1|
第2列と第3列を第1列に加える。
=|1+x+x−11+x1+x−11+x+x−1−11+x1+x+x−11+x−1−1|
=(1+x+x−1)|11+x1+x−11−11+x11+x−1−1|
第2行-第1行 第3行-第1行
=(1+x+x−1)|11+x1+x−10−2−xx−x−10x−1−x−2−x−1|
=(1+x+x−1){(2+x)(2+x−1)+(x−x−1)2}
また,
(2+x)(2+x−1)+(x−x−1)2
=4+2x−1+2x+1+x2−2+x−2=x2+1x2+2x+2x−1+3
=(x+1x)2−2+2(x+1x)+3=(x+x−1+1)2
|A|=(x+x−1+1)3
A−1=1|A|(¯A11¯A21¯A31¯A21¯A22¯A23¯A31¯A32¯A33)
=1|A|( 1−(1+x)(1+x−1)1+x+(1+x−1)2(1+x)2+1+x−11+x−1+(1+x)21−(1+x)(1+x−1)1+x+(1+x−1)2(1+x−1)2+1+x1+x−1+(1+x)21−(1+x)(1+x−1))
=1|A|(−(1+x+x−1)(1+x+x−1)(1+x−1)(1+x+x−1)(1+x)(1+x+x−1)(1+x)−(1+x+x−1)(1+x+x−1)(1+x−1)(1+x+x−1)(1+x−1)(1+x+x−1)(1+x−1)−(1+x+x−1))
=1(1+x+x−1)2(−1(1+x−1) 1+x1+x−1(1+x−1)(1+x−1)1+x−1)・・・答え
同じカテゴリー「数検1級の線形代数」の一覧
線形代数43(逆行列を求めるのに分数の計算を回避する方法)
掃出し法で逆行列を求めると必ず計算ミスをする人は必見 あくまでも掃き出し法でする場合 [math]A=\begin{pmatrix […]