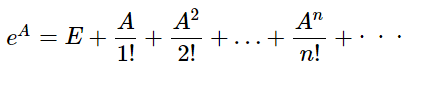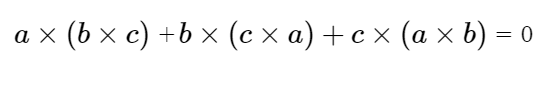ここで勉強すれば数学検定1級の壁は超えられるか。
MENU
数学検定1級の壁 TOP > 数検1級の線形代数 > 行列17の解説 (行列の内積)
行列17の解説 (行列の内積)

(13−1−2),(−2231) の2つ正方行列のなす角 θ を求める。
A=(13−1−2),B=(−223−1) とすると
tAB=(1−13−2)(−2231)=(−53−128)となる。
(A,B)=tr(tAB)=−5+8=3
tAA=(1−13−2)(13−1−2)=(25513)
tBB=(−232−1)(−223−1)=(13−7−75)
(A,A)=tr(tAA)=2+13=15
(B,B)=tr(tBB)=13+5=18
cosθ=(A,B)√(A,A)⋅(B,B)=3√15⋅√18=1√30・・・答え
同じカテゴリー「数検1級の線形代数」の一覧
線形代数43(逆行列を求めるのに分数の計算を回避する方法)
掃出し法で逆行列を求めると必ず計算ミスをする人は必見 あくまでも掃き出し法でする場合 [math]A=\begin{pmatrix […]