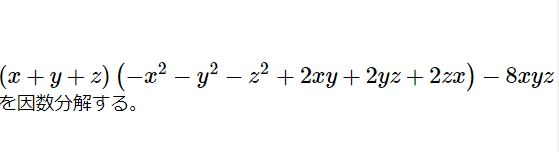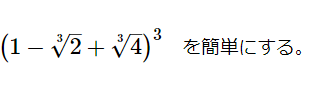ここで勉強すれば数学検定1級の壁は超えられるか。
MENU
数学検定1級の壁 TOP > 数検1級の式・方程式など > 無理数を含んだ式の展開(式・方程式17)
無理数を含んだ式の展開(式・方程式17)

(1−3√2+3√4)3 を簡単にする。
3√2=x,3√4=223=x2とおいて
(1−3√2+3√4)3=(1−x+x2)3
=(1−x)3+3(1−x)2x2+3(1−x)x4−x6
=x6−3x5+6x4−7x3+6x2−3x+1
=(x6−7x3+1)+(−3x5+6x4+6x2−3x)
x3=2より
=(x3)2−7x3+1−3⋅2x2+6⋅2⋅x+6x2−3x
=4−7×2+1−6x2+12x+6x2−3x
=−9+9x=−9+93√2=9(3√2−1)
9(3√2−1)・・・答え
同じカテゴリー「数検1級の式・方程式など」の一覧
3次方程式3の解と係数の関係の応用編(式・方程式20)
[math]a^{3}+b^{3}+c^{3}-3abc=\left( a+b+c\right) \left( a^{2}+b^{2}+c^{2}-cb-bc-ca\right)[/ma […]
x,y,zに関する3次式の因数分解(式・方程式16)
[math]xy\left( x^{2}-y^{2}\right) +yz\left( y^{2}-z^{2}\right) +zx\left( z^{2}-x^{2}\r […]