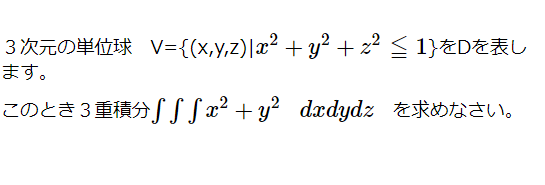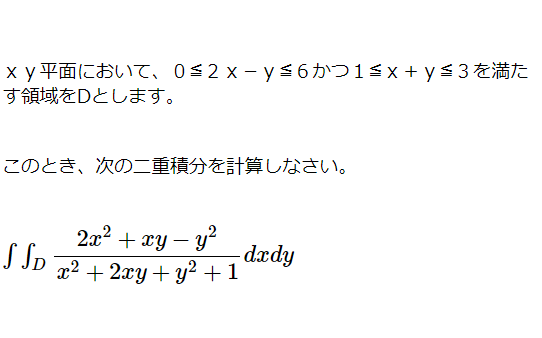ここで勉強すれば数学検定1級の壁は超えられるか。
MENU
数学検定1級の壁 TOP > 数検1級の重積分 > 重積分13の解説 (式に絶対値がはいっている2重積分)
重積分13の解説 (式に絶対値がはいっている2重積分)

平面上の領域D{(x,y)| 0≦x≦1,0≦y≦1 }のとき、
∫∫D|x−y|−23dxdy の2重積分を解く。

x≧yの場合(A)
∫∫(x−y)−23dydx=∫10dx∫x0(x−y)−23dy=∫10dx[−3(x−y)13]y=xy=0
=∫103x13dx=3[34x43]10=94
y≧xの場合(B)
=∫∫(y−x)−23dxdy=∫10dy∫y0(y−x)−23dx
=∫10dy[−3(y−x)13]x=yx=0=∫103y13dy
=3[34y43]10=94
∫∫D|x−y|−23dxdy=94+94=92
92・・・答え
同じカテゴリー「数検1級の重積分」の一覧
重積分14の解説(三角関数の2重積分)
重積分 予想問題 [math]D=\left\{ \left( x,y\right) \left| \right| y\leqq x\leqq y^{2},1 […]
重積分13の解説 (式に絶対値がはいっている2重積分)
平面上の領域D{(x,y)| 0≦x≦1,0≦y≦1 }のとき、 [math]\int \int _{D}\left| x-y\right| ^{-\dfrac {2}{3 […]
重積分12の解説 (円筒座標変換による重積分)
3次元の単位球 V={(x,y,z)|x2+y2+z2≦1}をDを表します。 このとき3重積分[math]\int \int \in […]
変数変換の二重積分(重積分11)
xy平面において、0≦2x-y≦6かつ1≦x+y≦3を満たす領域をDとしたとき、 [math]\int \int _{D}\dfrac {2x^{2}+xy- […]