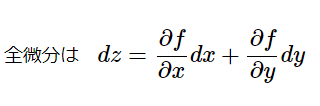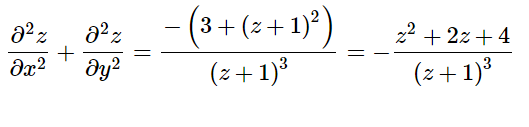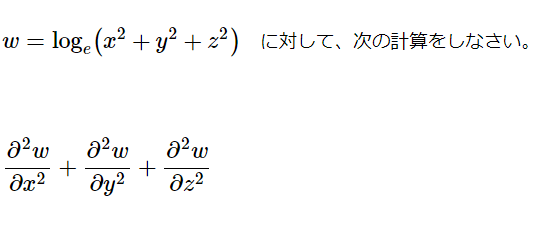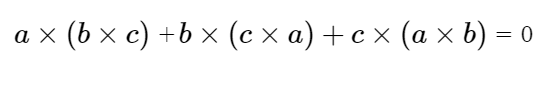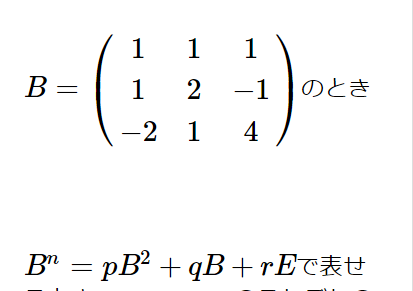ここで勉強すれば数学検定1級の壁は超えられるか。
MENU
数学検定1級の壁 TOP > 数検1級の微分 > 逆タンジェントの微分(微分3)
逆タンジェントの微分(微分3)

f(x)=tan−1(secx)
f′(x)=11+sec2x⋅(secx)′
f′(x)=11+sec2x⋅sinxcos2x
f′(π4)=11+2⋅1√212=23√2=√23・・・答え
同じカテゴリー「数検1級の微分」の一覧
微分17(2変数関数のマクローリン展開)
2変数のマクローリンの定理 [math]Df=\left( h\dfrac{\partial }{\partial x}+k\dfrac{\partial }{\parti […]