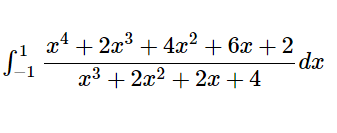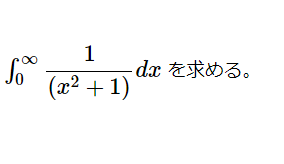ここで勉強すれば数学検定1級の壁は超えられるか。
MENU
数学検定1級の壁 TOP > 数検1級の積分 > 積分2の解説
積分2の解説

∫∞01(x2+1)4dx を求める。
x=tanθ⇒x2+1=1cos2θ,dx=1cos2θ
x:0→∞ θ:0→π2
∫∞01(x2+1)4dx=∫π20cos8θcos2θdθ
=∫π20cos6θdθ=56⋅34⋅12⋅π2=532π・・・答え
参考事項
In=∫π20cosnθdθ
nが偶数のとき
In=n−1n⋅n−3n−2−⋅…12⋅π2
nが奇数のとき
In=n−1n⋅n−3n−2−…67⋅45⋅23