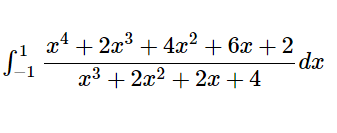ここで勉強すれば数学検定1級の壁は超えられるか。
MENU
数学検定1級の壁 TOP > 数検1級の積分 > 積分1の解説
積分1の解説

∫10(cos−1x)2dx
を求める。
cos−1x=y→cosy=x
−siny⋅dy=dx
x:0→1⇒y:π2→0
∫10(cos−1x)2dx=∫0π2y2siny⋅dy
=[cosy⋅y2]0π2−2∫0π2cosy⋅ydy
=−2∫0π2cosy.ydy
=−2([y⋅siny]0π2+[cosy]0π2)
=−2×((0−π2)+(1−0))=π−2・・・答え