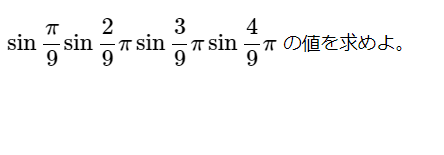ここで勉強すれば数学検定1級の壁は超えられるか。
MENU
数学検定1級の壁 TOP > 数検1級の複素数 > 複素数の2重根号(複素数13)
複素数の2重根号(複素数13)

iは虚数単位とします。√1+√3i+√1−√3iを簡単にしなさい。ただし、外側の平方根はどちらも実数部が正の値をとるものとします。
z=√1+√3i+√1−√3iとおくと、
z2=(√1+√3i)2+(√1−√3i)2+2√1+√3i√1−√3i
=2+2√12+3=6
z2=6⇒z=±√6
z>0⇒z=√6
√6・・・答え
別解
√1+√3i=√2−√1+√3i2=√2√(cosπ3+isinπ3)
√1−√3i=√2⋅√1−√3i2=√2⋅√cosπ3−isinπ3
√1+√3i+√1−√3i=√2(cosπ6+cosπ6+isinπ6−isinπ6)=2√2⋅cosπ6
したがって
√1+√3i+√1−√3i=2×√2×√32=√6
√6・・・答え
同じカテゴリー「数検1級の複素数」の一覧
正弦4つの積(複素数17)
[math]\sin \dfrac{\pi }{9} \sin \dfrac{2}{9}\pi \sin \dfrac{3}{9}\pi \sin \dfrac{4}{9}\pi […]
複素数の極形式の応用(複素数15)
次の計算をしなさい。ただし、iは虚数単位を表します。 [math]\dfrac {\left( 1-i\right) ^{11}}{\left( -\sqrt {3}+i\ […]